Home › Behaviors Popularized

How To Find Interval Of Increase: A Comprehensive Guide

AuraInsider November 30, 2024

Understanding how to find the interval of increase is crucial for students and professionals alike in the realms of mathematics and data analysis. This concept plays a significant role in calculus, specifically when dealing with functions and their graphical representations. In this article, we will delve deep into the methods and principles that govern finding intervals of increase for various functions.

We will explore not just the mathematical procedures, but also practical applications of these intervals in real-world scenarios. By the end of this guide, you will have a clear understanding of how to determine intervals of increase, supported by examples, graphs, and expert insights.

Whether you are a student preparing for an exam or a professional looking to brush up on your skills, this article will provide you with the knowledge and tools you need. Let’s embark on this mathematical journey together!

What is Interval of Increase?
Importance of Intervals of Increase
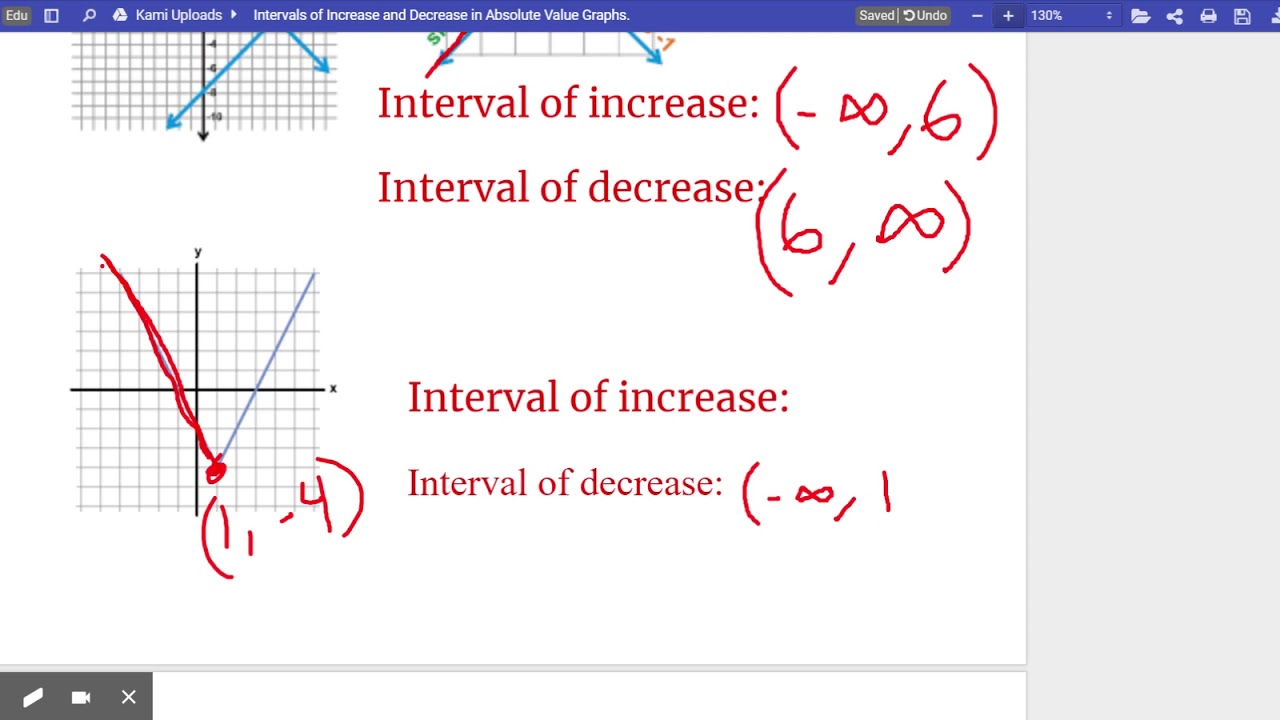
How to Find Intervals of Increase
Examples of Finding Intervals of Increase
Graphical Interpretation
Common Mistakes to Avoid
Applications in Real Life
Conclusion

What is Interval of Increase?

The interval of increase refers to the range of values for which a function rises as the input values increase. In mathematical terms, if a function \( f(x) \) is increasing on an interval \( (a, b) \), it means that for any two points \( x_1 \) and \( x_2 \) within that interval, if \( x_1 < x_2 \), then \( f(x_1) < f(x_2) \).

Understanding the Concept

To fully grasp the concept of intervals of increase, it's important to visualize how functions behave. Functions can be categorized as:

Increasing: The function goes up as you move from left to right.
Decreasing: The function goes down as you move from left to right.
Constant: The function remains flat.

Importance of Intervals of Increase

Understanding intervals of increase is essential for several reasons:

Optimization: Identifying where a function is increasing helps in optimization problems.
Graph Analysis: Knowing the intervals of increase can help in sketching graphs accurately.
Real-world Applications: Many real-world phenomena can be modeled using increasing functions.

How to Find Intervals of Increase

There are systematic steps to determine the intervals of increase for a given function:

Step 1: Find the Derivative

The first step is to compute the derivative of the function \( f(x) \). The derivative, denoted as \( f'(x) \), provides information about the slope of the function at any given point.

Step 2: Set the Derivative to Zero

Next, set the derivative equal to zero to find critical points. This can be done using the equation:

\( f'(x) = 0 \)

Step 3: Analyze the Sign of the Derivative

To determine where the function is increasing, analyze the sign of the derivative in the intervals defined by the critical points. If \( f'(x) > 0 \), the function is increasing in that interval; if \( f'(x) < 0 \), it is decreasing.

Step 4: Write the Intervals

Finally, summarize the intervals where the function is increasing. These intervals can be expressed in interval notation.

Examples of Finding Intervals of Increase

Let’s consider a couple of examples to illustrate how to find intervals of increase.

Example 1: Simple Polynomial Function

For the function \( f(x) = x^2 - 4x + 3 \):

Step 1: Find the derivative: \( f'(x) = 2x - 4 \).
Step 2: Set the derivative to zero: \( 2x - 4 = 0 \Rightarrow x = 2 \).
Step 3: Analyze the sign of the derivative: For \( x < 2 \), \( f'(x) < 0 \) (decreasing); for \( x > 2 \), \( f'(x) > 0 \) (increasing).
Step 4: The interval of increase is \( (2, \infty) \).

Example 2: Trigonometric Function

For the function \( f(x) = \sin(x) \):

Step 1: Find the derivative: \( f'(x) = \cos(x) \).
Step 2: Set the derivative to zero: \( \cos(x) = 0 \Rightarrow x = \frac{\pi}{2} + n\pi \) (where n is an integer).
Step 3: Analyze the sign of the derivative between critical points.
Step 4: The function is increasing in intervals like \( (2n\pi, (2n+1)\pi) \).

Graphical Interpretation

Graphing the functions can provide a visual representation of the intervals of increase. Here are some key points:

Look for regions where the graph slopes upwards.
Identify critical points where the slope changes from positive to negative.
Use graphing tools or calculators to visualize complex functions.

Common Mistakes to Avoid

When finding intervals of increase, be mindful of the following common mistakes:

Overlooking critical points.
Misinterpreting the sign of the derivative.
Forgetting to test intervals between critical points.

Applications in Real Life

Intervals of increase have practical applications in various fields:

Economics: Analyzing profit trends.
Physics: Understanding motion and acceleration.
Biology: Modeling population growth.

Conclusion

In conclusion, understanding how to find the interval of increase is a valuable skill that can greatly enhance your analytical capabilities. By following the steps outlined in this article, you can confidently determine where functions are increasing.

We encourage you to practice with various functions and share your findings in the comments below. If you found this article helpful, please consider sharing it with others who may benefit from this knowledge!

Call to Action

Leave your thoughts in the comments, share this article, or check out our other articles for more insights into mathematical concepts!

Thank you for reading, and we look forward to seeing you back here for more informative content!

Article Recommendations

Details